Using decimals, fractions & % to help measure probability

Lead In:

We began by discussing our central idea and what it means to us. This led to some great chats about how we use probability in our lives

° we use probability all the time in our daily lives even though we might not be so conscious of that thinking.  We discussed an idea of when we cross a road and we see an old, run-down car approaching at 50km/hr and a red Ferrari also approaching at 50 km/hr, we tend to use probability to think that the chances the old car will run us down is lower than the Ferrari.  We are sub-consciously thinking about probability all the time.

° weather forecasting is probability

° casino gambling became a hot topic: how do Casinos make money when people are always winning at them.  We discussed the expression ' The house always win' and some shared how they heard casinos pipe in extra oxygen to keep people awake and sell cheap food etc. Interesting chat. 

° thinking about what sort of sandwiches mum had made me for lunch today



                                 


We looked at our TV game show measurements of probability from yesterday (see previous blog) and discussed what helped us to solve the issues we were having over which had the higher probability of winning:   converting them all to fractions didn't help us much,but when we converted to decimals and later percentages, we could determine which games had a higher probability compared to others.

To help reinforce the understanding of how we can use decimals & percentages to measure probability, we began with metre rulers.   

At one end we labelled:   Certain  100%

At the other end:  Impossible 0%

Partners added other words we can use to measure the likelihood of a chance event occurring. After generating a list of words and placing them along the ruler, they then assigned a decimal and a percentage to each word. 

Combining all our ideas, our measurement of probability line ended up looking like this:

With this understanding, we were ready to play our TV game show activity again, but this time the probability games were a bit more challenging than yesterday's:



     You are on a TV game show.

You have a choice of 4 games to play to win a million francs.

Which game should you choose to play and why?

Game A	Game B
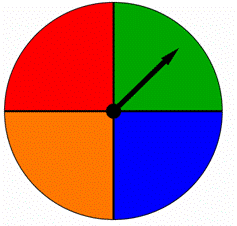
Flip 3 coins and if they land with at least 1 head and 1 tail, you win.	Spin green on this spinner twice in a row with 2 spins only and you win.
Game C	Game D
Pull a black number card from a pack of cards (shuffled & without jokers).	Roll 2 dice if they land on “lucky” 7, you win.

In partners, we tried our own strategies to work out the probabilities. Some of us wanted to test out the probability theories they had formed.Such a great idea! Watching the maths come alive!

After trying to measure the probability of winning each game with partners, we started sharing the orders we ranked the games from the highest to lowest probability of winning.  
















The heads and tails game again caused a split in the class of what the probability was.  So we created a 'mathematical fight of the century'. In one corner we had the agreerers and in the other the disagreers.

Both sides explained why they thought their probability was right.  Whilst defending their theory, one team actually disproved their original idea. :)


We started by using a fractional form:   X in X chance for each.

Can we compare the probabilities?

-No

What should we do?

- Convert them to a percentage or decimal

We voted on converting them all to a % and came up with the following:

Someone had suggested earlier that whoever gets the probabilities correct should be able to play the game to try to win the million francs.  Great, fun idea.

The game with the highest probability was Game A:

Flip 3 coins and if they land with at least 1 head and 1 tail, you win.

Three partners competed.  The odds of winning were pretty good at 3 in 4.

We were so shocked when the second partners actually flipped three tails!

Wow! They were out.

The other two teams both won a million francs.

To make it interesting they had a chance of double or nothing. Walk away with a million francs or play again to risk doubling. 

The first partners took their million francs and walked away as winners.

The other partner group however stayed to double.  Tense times.

I kid you not- the second flip they did for 2 million francs landed on 

heads, heads, heads.

They lost.

This is why we shouldn't gamble- an important life lesson was just demonstrated.

One student called out:  The house always wins!

And that seemed the best way to wrap up today's learning about probability.